3.3 Portfolio investment rules
We shall now present the major characteristics of the DPPI
that allow us to significantly improve the rating of the basic CPPI. The DPPI
indeed capitalizes on several structural features and investment rules in order
to achieve the target rating of Aa3 over a time horizon of 10 years.
3.3.1 Dynamic leverage function
The Target Notional Exposure at time t, noted TNE(t), is no
longer a constant multiple of the Reserve at time t, R(t), but a more
complicated function designed to take advantage of various market conditions.
For doing so, we need to define intermediary variables.
Duration of a CDS contract in a simplified intensity model
The duration D of a CDS contract of constant market spread s
and tenor T years with coupons being paid every Ät year, with
ÄtT := p ? N is given by the following formula:
p
E
i=1
D=
ÄtB(0, iÄt)S0(iÄt)
where we assume that the survival function S0(t) := 1 -
P(ô = t) is continuous,
differentiable and solves the following
ordinary differential equation with ë(t) ? R+*:
~ S0 0(t) + ë(t)S0(t) = 0
?t ? R+*,
S0(0) = 1
As a result, S0(t) = e-f0 t ë(s)ds. A way to empirically
determine the function ë is to assume that ë is piecewise constant
between all liquid tenors and to use the CDS valuation equation (1.1) to
determine ë recursively: the first step will be to determine ë(T1)
where T1 is the shortest tenor, whith ë(T1) = s(T 1)
1-R after simplifying equation (1.1) with T = T1. The second
step is to extend the maturity of equation (1.1) from T1 to T2, and express
S0(T1) and S0(T2) as a function of respectively ë(T1) and ë(T2). From
that equation, we infer ë(T2), and so on and so forth. R can then be taken
equal to 40% as as market convention and the spread s(Ti) can be read from
market quotations.
Target Notional Exposure function
We easily generalize the initial duration D of a CDS at time t
= 0 with tenor T to the duration function D(t) at time t = T and define the
average duration function D(t) of all long CDS positions in portfolio at time t
by simply taking the weighted arithmetic average of the durations of all single
CDS in portfolio. We further introduce the weighted average rating-dependent
risk function SR(t), which is homogeneous to a CDS spread:
PN n=1 An(t)C(Rn(t))
?t ? [0,T], SR(t) :=
PN n=1 An(t)
where C is an increasing mapping function from integer rating
categories {1, .., 18} to [0, 1] and where An(t) denotes the weight
of obligor n at time t expressed in units of initial notional A (>N n=1
An(t) = A).
We can then describe our dynamic target multiplier TM(t)
function:
1
?t ? [0, T ], T M(t) :=
DR + SR(t)D(t)
where DR is a risk parametre accounting for a portfolio average
default risk.
We then define the average 5-year market spread of the
portfolio at time t, S(t), by simply computing the weighted average 5-year
market spread of all obligors. We then define the piecewise constant
opportunity leverage mapping function OL(t) that maps the weighted average
5-year market spread S(t) with the leverage factor OL(S(t)).
We further introduce two path-dependent multiplying functions,
bu(t) and bd(t), that act respectively as exposure boost-up and
boost-down features:
I bu if t = tu and
max{v?[t-tu,t]}(S(v)) - min{v?[t-tu,t]}(S(v)) =
Äsu bu(t) = 1 if not
{ bd if t = td and S(t) - S(t - td) = Äsd
bd(t) = 1 if not
where (bu, tu, Asu,bd,td,
Asd) are ad-hoc structural parametres. The rationale for introducing
bu(t) and bd(t) is to make the structure proactive in both low and
high volatility spread environments.
Hence we can define the Target Notional Exposure function:
Definition 7. Target Notional Exposure
With notations introduced earlier, the Target Notional Exposure
function behaves according to the following formula:
?t ? [0, T], TNE(t) := bu(t)bd(t) min {OL(t)A, T
M(t)R(t)} Notional Exposure function
As a result, the exposure of the CDS portfolio is either
increased or decreased depending on whether the current Notional Exposure NE(t)
is far enough from the Target Notional Exposure TNE(t):
Definition 8. Notional Exposure
With notations introduced earlier, the Notional Exposure function
behaves according to the following formula:
?t ? [0, T],
NE(t+) = NE(t-)1{ TNE(t)
+ TNE(t)1{ TNE(t)
NE(t
?[(1-lb),(1+lu)]}

NE(t-) /?[(1-lb),(1+lu)]}
where lu ? [0, 1] and ld ? [0,1] are upper and lower
leverage readjustment bounds. 3.3.2 Deferred coupons
One of the core features of the DPPI is its ability to defer
interest payments to
investors when its NPV is deemed not to be high enough.
The following recursive
formula gives the potential interest payment to be
made on any coupon payment
date kAt, with TÄt ? N* and k ? {1, ..,
T Ät}.
Definition 9. Deferred Interests
Assume t ? {0, At, 2At,..,T}. Let EUR(t,t + At) denote the
EURIBOR rate observed in t with tenor At. Let us introduce the flow
variable:
x(t) := [R(t) - NE(t)u(t)]+
where u(t) is a real parametre ? [0,1]. We then define:
(IP(t), DI(t-),
DI(t+)){t?{0,Ät,2Ät,..,T}
as being respectively the interest payment on date t, the
deferred interest balance just
before t and right after t. The
following recursive formula relates all three variables:
(IP (0), DI(0-), DI(0+)) = (0,0,0)
?t ? {At, 2At, .., T},
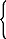
IP(t) = min {DI(t-) + AAtEUR(t - At, t), x(t)}
DI(t+) = DI(t-) + AAtEUR(t - At, t) -
1{IP(t)=0}IP(t) DI(t-) = DI(t+ - At)(1 +
AtEUR(t - At, t))
| 


