II.1 Exchange rate and fundamentals
a) Flexi-price Monetary model
The model concentrates on the current account and assumes
prices are flexible and output is exogenous, determine by the supply side of
the economy. It's relies on the PPP condition and a stable demand for money
function. The logarithm of the demand for money may be assumed to depend on the
log of real income, Y, the price level, P, and the level of the interest rate,
r
  (Domestic equilibrium) (2.3) (Domestic equilibrium) (2.3)
  (Foreign equilibrium) (2.4) (Foreign equilibrium) (2.4)
Where ? is a coefficient parameter of real income and ë the
coefficient of the interest rate. In this model the domestic r is exogenous
because of the assumption of perfect capital mobility and a zero expected
change in the exchange rate. The equilibrium in traded exchange market ensues
when prices in a common currency are equalised; by using the PPP condition:
  (2.5) (2.5)
b) Dornbusch overshooting model
In 1976, Dornbusch model begin with a description of the main
behavioural assumption. The uncovered interest parity relationship expresses
the conditions for equilibrium. Foreign exchange speculators investing abroad
expect a return of   +u%, where +u%, where   the foreign interest rate and u is is the expected appreciation of
foreign currency (depreciation in domestic currency). the foreign interest rate and u is is the expected appreciation of
foreign currency (depreciation in domestic currency).   (2.6) (2.6)
the expectation about the exchange rate are assumed to be
regressive; if the actual rate lies below the long run equilibrium rate, s,
therefore the expectation of actual rate to rise towards the long-run(Keith,
p293); 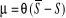   (2.7) (2.7)
The rational expectation in this formula allows having a
correct expectation:
 (Equation in the money market) (Equation in the money market)
 (Good market) (Good market)
Where the first term represents the impact of real exchange
rate on net trade volumes, the second (-är) is the invest schedule, the
third term ( Y) the consumption function and expenditure effects on imports and the
final term ( Y) the consumption function and expenditure effects on imports and the
final term ( ') exogenous demand factors such as government expenditure. ') exogenous demand factors such as government expenditure.
c) Frankel real interest differential model
In 1979 Jeffrey Frankel provides a model for analysing the
impact of change in the interest rate on the exchange rate and he refers to
this as «the real interest differential model». This model assumes
uncovered arbitrage but modifies the Dornbusch expectation equation for the
exchange rate by adding a term reflecting relative expected secular
inflation the expectation equation is(Keith p295): the expectation equation is(Keith p295):
And an uncovered interest parity yield is: 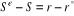
The term  is the expected rate of depreciation which depends upon the deviation
of the exchange rate from its equilibrium value. If is the expected rate of depreciation which depends upon the deviation
of the exchange rate from its equilibrium value. If , the expected rate of depreciation is given by the expected inflation
differential. , the expected rate of depreciation is given by the expected inflation
differential.
d) Noise Traders model
It have been noted that the risk neutrality and rational
expectation assumptions are not consistent with the empirical results on
forward rate unbiased and speculation in the spot market through the uncovered
interest parity condition. The irrational traders probably do influence spot
rates but such behaviour, based on results from chartists expectation, are
likely to have only a short run impact on freely floating spot rates and
chartists behaviour is unlikely to be destabilising, independently of other
trader's behaviour. They may drive a value of the asset away from its
fundamental value, and creates an opportunity for arbitrage for the rational
investor.
| 


